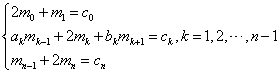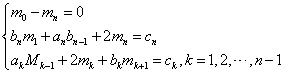三次样条插值 C#代码实现

一、样条函数的定义

样条函数属于分段光滑插值，他的基本思想是，在由两相邻节点所构成的每一个小区间内用低次多项式来逼近，并且在各结点的连接处又保证是光滑的(即导数连续)。

设在区间[a,b]上给定一组结点X:

,和一组对应的函数值。若函数S(x)满足下列条件：

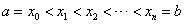
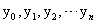
(1)在每一个子区间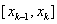(k=1,2,…n)上，S(x)是一个不超过三次的多项式。

(2)在每一个结点上满足S(xi)=yi,i=0,1,…,n。

(3)S(x)在区间[a,b]上为二次连续可微函数。

则称S(x)为结点X上插值与Y的三次样条插值。

二、三次样条函数的构造

在工程上，构造三次样条插值函数通常有两种方法：一是以给定插值结点处得二阶导数值作为未知数来求解，而工程上称二阶导数为弯矩，因此，这种方法成为三弯矩插值。二是以给定插值结点处得一阶导数作为未知数来求解，而一阶导数右称为斜率，因此，这种方法称为三斜率插值。

三斜率插值法

根据定义，三次样条函数在插值结点处一阶导数应存在。因此设各结点处的一阶导数为：

。利用两点埃尔米特插值公式，就可以得到样条插值函数S(x)在子区间上的表达式为：

 (1)

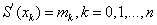
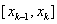
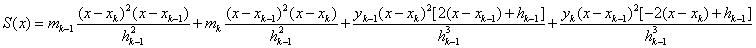
其中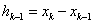：。由此可知只要确定各结点的一阶导数值mk(k=0,1,2….n)，则各子区间上的三次样条插值函数S(x)也就确定了。

由于S(x)在区间[a,b]上的二阶导数是连续的，即在各结点的左右两子区间上的S(x)虽然不同，但在连接点的二阶导数存在，即在连接点处的二阶左导数与二阶右导数相等：。分别求子区间[xk-1,xk]右端点xk上的二阶左导数，以及子区间[xk,xk+1]左端点xk上的右导数，即可得到：

，(2)与 ，(3) 整理后可得：，k=1,2,…n-1,(4)。

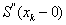
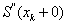
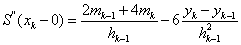
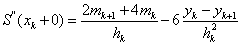
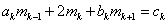
其中，并且ak+bk=1。由于有n+1个插值点，因此需要确定n+1个m(m0,m1,…,mn)。而现在方程组只有n-1个方程(公式(4))，因此还需要另外补充两个条件财能唯一确定n+1个m。在实际应用中，这两个条件可以根据实际的物理意义所给出的边界条件来得到。实际问题中常用的三种边界条件如下：

(1)给定区间两个端点处的一阶导数值，即

则根据方程组(4)可得到新的方程组：

方程组的系数矩阵为，可知此矩阵为三对角矩阵。因此方程组可用追赶法求解。

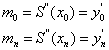
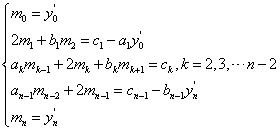
解出mk后，即得到各结点的一阶导数值，将mk带入各结点的二阶导数值得表达式公式(2)或(3)可求得各结点的二阶导数值，将mk带入各区间上S(x)的表达式公式(1)即可得到各子区间上的三次样条插值函数S(x)。

(2)给定区间两个端点处得二阶到数值，即

，由此可得两个补充方程，其中。与公式(4)联立可得如下方程组:

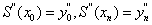
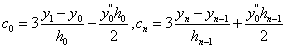
(3)插值函数为周期函数

yn=y0，且，由此可得两个补充方程为，其中

，并且an+bn=1。最后可得方程组：

在此给出第一种边界条件下三次样条插值的C#算法实现：

1、实现三次样条插值封装的类：

/// <summary>
    /// 插值
    /// </summary>
    public static class SplineMath
    {
        /// <summary>
        /// 三次样条插值
        /// </summary>
        /// <param name="points">排序好的数</param>
        /// <param name="xs">需要计算的插值点</param>
        /// <param name="chf">写1</param>
        /// <returns>返回计算好的数值</returns>
        public static double[] SplineInsertPoint(PointClass[] points, double[] xs, int chf)
        {
            int plength = points.Length;
            double[] h = new double[plength];
            double[] f = new double[plength];
            double[] l = new double[plength];
            double[] v = new double[plength];
            double[] g = new double[plength];

            for (int i = 0; i < plength - 1; i++)
            {
                h[i] = points[i + 1].x - points[i].x;
                f[i] = (points[i + 1].y - points[i].y) / h[i];
            }

            for (int i = 1; i < plength - 1; i++)
            {
                l[i] = h[i] / (h[i - 1] + h[i]);
                v[i] = h[i - 1] / (h[i - 1] + h[i]);
                g[i] = 3 * (l[i] * f[i - 1] + v[i] * f[i]);
            }

            double[] b = new double[plength];
            double[] tem = new double[plength];
            double[] m = new double[plength];
            double f0 = (points[0].y - points[1].y) / (points[0].x - points[1].x);
            double fn = (points[plength - 1].y - points[plength - 2].y) / (points[plength - 1].x - points[plength - 2].x);

            b[1] = v[1] / 2;
            for (int i = 2; i < plength - 2; i++)
            {
                // Console.Write(" " + i);
                b[i] = v[i] / (2 - b[i - 1] * l[i]);
            }
            tem[1] = g[1] / 2;
            for (int i = 2; i < plength - 1; i++)
            {
                //Console.Write(" " + i);
                tem[i] = (g[i] - l[i] * tem[i - 1]) / (2 - l[i] * b[i - 1]);
            }
            m[plength - 2] = tem[plength - 2];
            for (int i = plength - 3; i > 0; i--)
            {
                //Console.Write(" " + i);
                m[i] = tem[i] - b[i] * m[i + 1];
            }
            m[0] = 3 * f[0] / 2.0;
            m[plength - 1] = fn;
            int xlength = xs.Length;
            double[] insertRes = new double[xlength];
            for (int i = 0; i < xlength; i++)
            {
                int j = 0;
                for (j = 0; j < plength; j++)
                {
                    if (xs[i] < points[j].x)
                        break;
                }
                j = j - 1;
                Console.WriteLine(j);
                if (j == -1 || j == points.Length - 1)
                {
                    if (j == -1)
                        throw new Exception("插值下边界超出");
                    if (j == points.Length - 1 && xs[i] == points[j].x)
                        insertRes[i] = points[j].y;
                    else
                        throw new Exception("插值下边界超出");
                }
                else
                {
                    double p1;
                    p1 = (xs[i] - points[j + 1].x) / (points[j].x - points[j + 1].x);
                    p1 = p1 * p1;
                    double p2; p2 = (xs[i] - points[j].x) / (points[j + 1].x - points[j].x);
                    p2 = p2 * p2;
                    double p3; p3 = p1 * (1 + 2 * (xs[i] - points[j].x) / (points[j + 1].x - points[j].x)) * points[j].y + p2 * (1 + 2 * (xs[i] - points[j + 1].x) / (points[j].x - points[j + 1].x)) * points[j + 1].y;

                    double p4; p4 = p1 * (xs[i] - points[j].x) * m[j] + p2 * (xs[i] - points[j + 1].x) * m[j + 1];
                    //         Console.WriteLine(m[j] + " " + m[j + 1] + " " + j);
                    p4 = p4 + p3;
                    insertRes[i] = p4;
                    //Console.WriteLine("f(" + xs[i] + ")= " + p4);
                }

            }
            //Console.ReadLine();
            return insertRes;
        }
    }

2、插值前需要对数据进行排序，需要使用PointClass类：

public class PointClass
    {
        public double x = 0;
        public double y = 0;
        public PointClass()
        {
            x = 0; y = 0;
        }
        //-------写一个排序函数，使得输入的点按顺序排列，是因为插值算法的要求是，x轴递增有序的---------
        public static PointClass[] DeSortX(PointClass[] points)
        {
            int length = points.Length;
            double temx, temy;
            for (int i = 0; i < length - 1; i++)
            {
                for (int j = 0; j < length - i - 1; j++)
                    if (points[j].x > points[j + 1].x)
                    {

                        temx = points[j + 1].x;
                        points[j + 1].x = points[j].x;
                        points[j].x = temx;
                        temy = points[j + 1].y;
                        points[j + 1].y = points[j].y;
                        points[j].y = temy;
                    }
            }
            return points;
        }
    }

3、具体实现：

private void btnCalcSpline_Click(object sender, EventArgs e)
        {
            double[] x = {-100,-90,-80,-70,-60,-50,-40,-30,-20,-10,0,10 ,20,30,40,50,60,70,80,90,100};
            double[] y = {9802,7922,6242,4762,3482,2402,1522,842,362,82,2,122,442,962,1682,2602,3722,5142,6562,8282,10202};
            PointClass[] points = new PointClass[x.Length];

            for (int i=0;i<x.Length;i++)
            {
                points[i] = new PointClass();
                points[i].x = x[i];
                points[i].y = y[i];

            }
            PointClass.DeSortX(points); //排序

            //double[] xs = { 0,1,80 };
            double[] xs = { Convert.ToDouble(txtX.Text) };
            try
            {
                double[] Y = SplineMath.SplineInsertPoint(points, xs, 1);
                txtY.Text = Y[0].ToString();
            }
            catch(Exception ex)
            {
                MessageBox.Show(ex.Message);
            }
        }

今天的文章三次样条插值 C#代码实现分享到此就结束了，感谢您的阅读，如果确实帮到您，您可以动动手指转发给其他人。