title: Array.sort和Collections.sort实现原理解析

date: 2017-02-13 19:22:01

tags: java基础

Arrays.sort和Collections.sort实现原理解析

1、使用

• 排序

2、原理

1. 事实上Collections.sort方法底层就是调用的array.sort方法，而且不论是Collections.sort或者是Arrays.sort方法，

2. 跟踪下源代码吧，首先我们写个demo

public static void main(String[] args) {
                 List<String> strings = Arrays.asList("6", "1", "3", "1","2");

                 Collections.sort(strings);//sort方法在这里

                 for (String string : strings) {

                     System.out.println(string);
                 }
             }

简单得不能再简单的方法了，让我们一步步跟踪

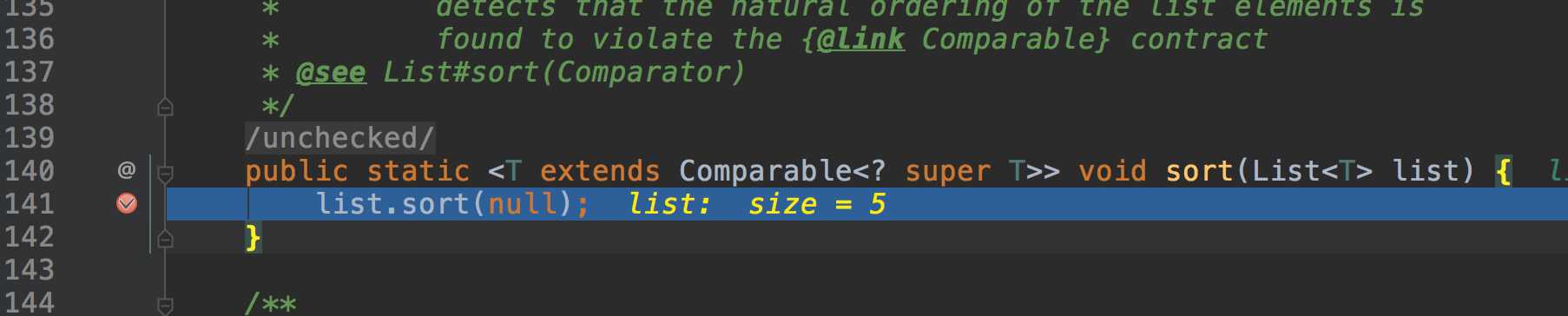
1. OK，往下面看，发现collections.sort方法调用的list.sort

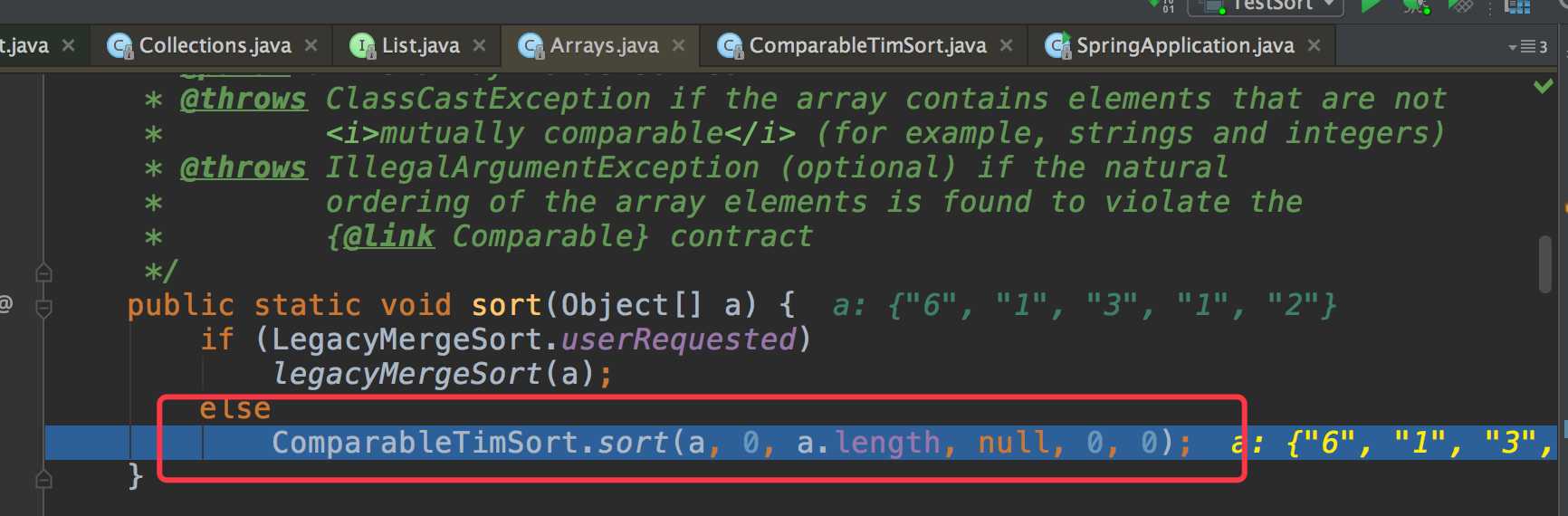
1. 然后跟踪一下，list里面有个sort方法，但是list是一个接口，肯定是调用子类里面的实现，这里我们demo使用的是一个Arrays.asList方法，所以事实上我们的子类就是arraylist了。OK，看arraylist里面sort实现，选择第一个，为什么不选择第二个呢？（可以看二楼评论，解答得很正确，简单说就是用Arrays.sort创建的ArrayList对象）

​

1. OK，发现里面调用的Arrays.sort(a, c); a是list,c是一个比较器，我们来看一下这个方法
   

我们没有写比较器，所以用的第二项，LegacyMergeSort.userRequested这个bool值是什么呢？
跟踪这个值，我们发现有这样的一段定义：

  > Old merge sort implementation can be selected (for
  >  compatibility with broken comparators) using a system property.
  >  Cannot be a static boolean in the enclosing class due to
  >  circular dependencies. To be removed in a future release.

  反正是一种老的归并排序，不用管了现在默认是关的

1. OK，我们走的是sort(a)这个方法，接着进入这个
   
2. 接着看我们重要的sort方法

static void sort(Object[] a, int lo, int hi, Object[] work, int workBase, int workLen) {
                 assert a != null && lo >= 0 && lo <= hi && hi <= a.length;

                 int nRemaining  = hi - lo;
                 if (nRemaining < 2)
                     return;  // array的大小为0或者1就不用排了

                 // 当数组大小小于MIN_MERGE(32)的时候，就用一个"mini-TimSort"的方法排序，jdk1.7新加
                 if (nRemaining < MIN_MERGE) {
                    //这个方法比较有意思，其实就是将我们最长的递减序列，找出来，然后倒过来
                     int initRunLen = countRunAndMakeAscending(a, lo, hi);
                     //长度小于32的时候，是使用binarySort的
                     binarySort(a, lo, hi, lo + initRunLen);
                     return;
                 }
                //先扫描一次array，找到已经排好的序列，然后再用刚才的mini-TimSort，然后合并，这就是TimSort的核心思想
                 ComparableTimSort ts = new ComparableTimSort(a, work, workBase, workLen);
                 int minRun = minRunLength(nRemaining);
                 do {
                     // Identify next run
                     int runLen = countRunAndMakeAscending(a, lo, hi);

                     // If run is short, extend to min(minRun, nRemaining)
                     if (runLen < minRun) {
                         int force = nRemaining <= minRun ? nRemaining : minRun;
                         binarySort(a, lo, lo + force, lo + runLen);
                         runLen = force;
                     }

                     // Push run onto pending-run stack, and maybe merge
                     ts.pushRun(lo, runLen);
                     ts.mergeCollapse();

                     // Advance to find next run
                     lo += runLen;
                     nRemaining -= runLen;
                 } while (nRemaining != 0);

                 // Merge all remaining runs to complete sort
                 assert lo == hi;
                 ts.mergeForceCollapse();
                 assert ts.stackSize == 1;
         }

1. 回到5，我们可以看到当我们写了比较器的时候就调用了TimSort.sort方法，源码如下

static <T> void sort(T[] a, int lo, int hi, Comparator<? super T> c,
                                  T[] work, int workBase, int workLen) {
                 assert c != null && a != null && lo >= 0 && lo <= hi && hi <= a.length;

                 int nRemaining  = hi - lo;
                 if (nRemaining < 2)
                     return;  // Arrays of size 0 and 1 are always sorted

                 // If array is small, do a "mini-TimSort" with no merges
                 if (nRemaining < MIN_MERGE) {
                     int initRunLen = countRunAndMakeAscending(a, lo, hi, c);
                     binarySort(a, lo, hi, lo + initRunLen, c);
                     return;
                 }

                 /** * March over the array once, left to right, finding natural runs, * extending short natural runs to minRun elements, and merging runs * to maintain stack invariant. */
           TimSort<T> ts = new TimSort<>(a, c, work, workBase, workLen);
                int minRun = minRunLength(nRemaining);
                do {
                    // Identify next run
                    int runLen = countRunAndMakeAscending(a, lo, hi, c);

                    // If run is short, extend to min(minRun, nRemaining)
                    if (runLen < minRun) {
                        int force = nRemaining <= minRun ? nRemaining : minRun;
                        binarySort(a, lo, lo + force, lo + runLen, c);
                        runLen = force;
                    }

                    // Push run onto pending-run stack, and maybe merge
                    ts.pushRun(lo, runLen);
                    ts.mergeCollapse();

                    // Advance to find next run
                    lo += runLen;
                    nRemaining -= runLen;
                } while (nRemaining != 0);

                // Merge all remaining runs to complete sort
                assert lo == hi;
                ts.mergeForceCollapse();
                assert ts.stackSize == 1;
      }

和上面的sort方法是一样的，其实也就是TimSort的源代码

3、总结

不论是Collections.sort方法或者是Arrays.sort方法，底层实现都是TimSort实现的，这是jdk1.7新增的，以前是归并排序。TimSort算法就是找到已经排好序数据的子序列，然后对剩余部分排序，然后合并起来

今天的文章Arrays.sort和Collections.sort实现原理解析分享到此就结束了，感谢您的阅读。