geometry-api-java 学习笔记（五）多边形 Polygons

A polygon is defined by a collection of rings. Each ring is a collection of contiguous line segments suchthat the start point and the end point are the same.

看下图，多边形的起点和终点相等。

Ring

Boundary and Rings

The boundary of a polygon is the collection of rings by which the polygon is defined. The boundarycontains one or more outer rings and zero or more inner rings. An outer ring is oriented clockwise whilean inner ring is oriented counterclockwise. Imagine walking clockwise along an outer ring. The area toyour immediate right is the interior of the polygon and to your left is the exterior.

Outer Ring

Similarly, if you were to walk counter-clockwise along an inner ring, the area to your immediateright is the interior of the polygon and to your left is the exterior.

Outer & Inner Ring

It is important to understand the boundary, interior and exterior of a geometry when using the variousoperators. The relational operators, for example, rely heavily on these concepts.

If a polygon has an inner ring, the inner ring looks like a hole. If the hole containsanother outer ring, that outer ring looks like an island.

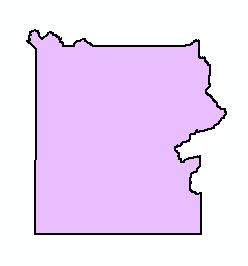1 outer ring, no inner rings	4 outer rings, no inner rings	1 outer ring, 1 inner ring	2 outer rings, 1 inner ring

Valid polygons

A valid polygon has no overlapping rings, no self-intersections except possiblyat vertices, no dangling segments and, in general, an arbitrary point can always beclassified unambiguously as either in the exterior, in the interior or on theboundary of the polygon. A valid polygon is said to be simple.See the Simplify operator for a more detailed specificationof simple polygons. Note that a simple polygon may not be OGC compliant. See the Simplify operator with OGC restrictions.

Examples

Let’s look at some examples of non-simple vs. simple polygons. The green circles are the vertices of the polygon,and the lavender colored area represents the interior of the polygon.

Non-simple polygons
Self-intersection	Self-intersection	Dangling segment	Dangling segment	Overlapping rings

Simple polygons
No self-intersection	Self-intersection at vertex	No dangling segment	No dangling segment	No overlapping rings

When drawing a polygon, use the even-odd fill rule. The even-odd fill rule will guarantee that the polygonwill draw correctly even if the ring orientation is not as described above for simple polygons.

JSON format

A polygon can be represented as a JSON string. A polygon in JSON format contains an array of ringsand an optional spatialReference. A polygon can also have boolean-valued hasM and hasZ fields. The default value is falsefor both the hasM and hasZ fields.

Each ring is represented by an array of points. Exterior rings are oriented clockwise, while interior ringsare oriented counter-clockwise. The order of the rings is irrelevant. The first point of each ring is always the same as the last point.Each point in a ring is represented as an array of numbers. See the description ofJSON multipoints for details on the interpretation of the point arrays.

An empty polygon is represented with an empty array for the rings field.

Syntax

{
  "hasZ" : true | false,
  "hasM" : true | false,
  "rings": [
             [[<x11>,<y11>,<z11>,<m11>],[<x12>,<y12>,<z12>,<m12>], ... ,[<x1j>,<y1j>,<z1j>,<m1j>]],
             ... ,
             [[<xn1>,<yn1>,<zn1>,<mn1>],[<xn2>,<yn2>,<zn2>,<mn2>], ... ,[<xnk>,<ynk>,<znk>,<mnk>]]
           ],
  "spatialReference" : {"wkid" : <wkid>}
}

2D Polygon

{
  "rings": [
             [[6453,16815],[10653,16423],[14549,5204],[-7003,6939],[6453,16815]],
             [[914,7992],[3140,11429],[1510,10525],[914,7992]]
           ],
  "spatialReference" : {"wkid" : 54004}
}

3D Polygon with Ms

Note that the third point does not have a z-value, and the second ring does not have any m-values.

{
  "hasZ" : true,
  "hasM" : true,
  "rings": [
             [[6453,16815,35,1],[10653,16423,36,2],[14549,5204,null,3],[-7003,6939,37,4],[6453,16815,35,1]],
             [[914,7992,30],[3140,11429,29],[1510,10525,28],[914,7992,30]]
           ],
  "spatialReference" : {"wkid" : 54004}
}

Empty Polygon

{"rings": []}

Creating a polygon

To create a polygon, we can use the Polygon class methods or one of the import operators.

Each of the code samples below creates a polygon with two outer rings and one inner ring.
The polygon looks like this:

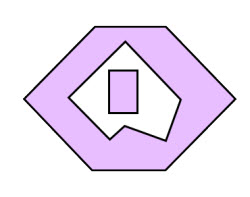Create this polygon

Polygon class methods

When using the Polygon class methods to create a polygon, the order in which the rings are createddoesn’t matter. What matters is the ring orientation. Remember, clockwise implies an outer ring whereascounter-clockwise implies an inner ring.

To begin each ring, we call the startPath method and then successive calls to thelineTo method. We don’t need to repeat the start point as the end point.

这里同样用到startPath和lineTo方法，不过不用重复把起点去做终点。

public static Polygon createPolygon1()
{
    Polygon poly = new Polygon();

    // clockwise => outer ring
    poly.startPath(0, 0);
    poly.lineTo(-0.5, 0.5);
    poly.lineTo(0.5, 1);
    poly.lineTo(1, 0.5);
    poly.lineTo(0.5, 0);

    // hole
    poly.startPath(0.5, 0.2);
    poly.lineTo(0.6, 0.5);
    poly.lineTo(0.2, 0.9);
    poly.lineTo(-0.2, 0.5);
    poly.lineTo(0.1, 0.2);
    poly.lineTo(0.2, 0.3);

    // island
    poly.startPath(0.1, 0.7);
    poly.lineTo(0.3, 0.7);
    poly.lineTo(0.3, 0.4);
    poly.lineTo(0.1, 0.4);

    return poly;
}

Import from JSON

As with the Polygon class methods, when using OperatorImportFromJsonto create a polygon the order in which the rings are created doesn’t matter. What matters is thering orientation. Unlike the Polygon class methods, the start point of each ring mustbe repeated to specify the end point.

The code shown below creates the same polygon as before, but notice that the inner ring that forms the holeis given before the outer ring. This was done just to drive home the point that the order of the ringsdoesn’t matter when the polygon is in JSON format.

static Polygon createPolygonFromJson() throws JsonParseException, IOException
{
    String jsonString = "{\"rings\":[[[0.5,0.2],[0.6,0.5],[0.2,0.9],[-0.2,0.5],[0.1,0.2],[0.2,0.3],[0.5,0.2]],"
                      + "[[0.0,0.0],[-0.5,0.5],[0.0,1.0],[0.5,1.0],[1.0,0.5],[0.5,0.0],[0.0,0.0]],"
                      + "[[0.1,0.7],[0.3,0.7],[0.3,0.4],[0.1,0.4],[0.1,0.7]]],"
                      + " \"spatialReference\":{\"wkid\":4326}}";

    MapGeometry mapGeom = OperatorImportFromJson.local().execute(Geometry.Type.Polygon, jsonString);

    return (Polygon)mapGeom.getGeometry();
}

Import from GeoJSON

Unlike the Polygon class methods and OperatorImportFromJson, when usingOperatorImportFromGeoJson to create a polygon the order in which the rings are givendoes matter. Within an array of rings, the outer ring is always first followed by zero or moreinner rings. However, the order of the arrays of rings doesn’t matter. The start point of eachring must be repeated to specify the end point.

The code shown below creates the same polygon as in the previous examples.

static Polygon createPolygonFromGeoJson() throws JsonParseException, IOException
{
    String geoJsonString = "{\"type\":\"MultiPolygon\","
                         + "\"coordinates\":[[[[0.0,0.0],[-0.5,0.5],[0.0,1.0],[0.5,1.0],[1.0,0.5],[0.5,0.0],[0.0,0.0]],"
                         + "[[0.5,0.2],[0.6,0.5],[0.2,0.9],[-0.2,0.5],[0.1,0.2],[0.2,0.3],[0.5,0.2]]],"
                         + "[[[0.1,0.7],[0.3,0.7],[0.3,0.4],[0.1,0.4],[0.1,0.7]]]],"
                         + "\"crs\":\"EPSG:4326\"}";

    MapGeometry mapGeom = OperatorImportFromGeoJson.local().execute(GeoJsonImportFlags.geoJsonImportDefaults, Geometry.Type.Polygon, geoJsonString, null);

    return (Polygon)mapGeom.getGeometry();
}

Import from WKT

As with OperatorImportFromGeoJson, when using OperatorImportFromWktto create a polygon the order in which the rings are given does matter. Within an array of rings,the outer ring is always first followed by zero or more inner rings. However, the order of the arraysof rings doesn’t matter. The start point of each ring must be repeated to specify the end point.

The code shown below creates the same polygon as in the previous examples, but notice that the outer ringthat forms the island is given first. This was done for illustrative purposes. It could have been given last,which probably would be more understandable to the reader.

static Polygon createPolygonFromWKT() throws JsonParseException, IOException
{
    String wktString = "MULTIPOLYGON (((0.1 0.7, 0.1 0.4, 0.3 0.4, 0.3 0.7, 0.1 0.7)),"
                     + "((0 0, 0.5 0, 1 0.5, 0.5 1, 0 1, -0.5 0.5, 0 0),"
                     + "(0.5 0.2, 0.2 0.3, 0.1 0.2, -0.2 0.5, 0.2 0.9, 0.6 0.5, 0.5 0.2)))";

    Geometry geom = OperatorImportFromWkt.local().execute(WktImportFlags.wktImportDefaults, Geometry.Type.Polygon, wktString, null);

    return (Polygon)geom;
}

Extracting Outer Rings

Many workflows require getting only the outer rings of a polygon.

The method getAllOuterRings extracts all the outer rings of a polygon. The call toOperatorSimplifyOGC makes sure there are no self-intersections, all outer rings areclockwise, and holes are counterclockwise. You can skip the call to OperatorSimplifyOGCif you trust your input polygon.

static List<Polygon> getAllOuterRings(Polygon polygon)
{
  Polygon simplePolygon = (Polygon)OperatorSimplifyOGC.local().execute(polygon, null, true, null);
  ArrayList<Polygon> rings = new ArrayList<Polygon>();
  int n = simplePolygon.getPathCount();
  for (int i = 0; i < n; i++)
  {
    if (simplePolygon.calculateRingArea2D(i) <= 0)
      continue;

    Polygon ring = new Polygon();
    ring.addPath(simplePolygon, i, true);
    rings.add(ring);
  }

  return rings;
}

The method getOutermostRings extracts the outermost rings, that is,only rings that are not contained inside of any hole.

static List<Polygon> getOutermostRings(Polygon polygon)
{
  List<Polygon> allOuterRings = getAllOuterRings(polygon);
  GeometryCursor outerRingsCursor = new SimpleGeometryCursor(allOuterRings);
  GeometryCursor unionCursor = OperatorUnion.local().execute(outerRingsCursor, null, null);
  Geometry unionPoly = unionCursor.next();

  // Holes could have been produced during the union, so rerun just in case.
  return getAllOuterRings((Polygon)unionPoly);
}

今天的文章geometry-api-java 学习笔记（五）多边形 Polygons分享到此就结束了，感谢您的阅读。